参与本项目,贡献其他语言版本的代码,拥抱开源,让更多学习算法的小伙伴们收益!
相对于贪心算法:跳跃游戏难了不少,做好心里准备!
给定一个非负整数数组,你最初位于数组的第一个位置。
数组中的每个元素代表你在该位置可以跳跃的最大长度。
你的目标是使用最少的跳跃次数到达数组的最后一个位置。
示例:
- 输入: [2,3,1,1,4]
- 输出: 2
- 解释: 跳到最后一个位置的最小跳跃数是 2。从下标为 0 跳到下标为 1 的位置,跳 1 步,然后跳 3 步到达数组的最后一个位置。
说明: 假设你总是可以到达数组的最后一个位置。
本题相对于55.跳跃游戏还是难了不少。
但思路是相似的,还是要看最大覆盖范围。
本题要计算最小步数,那么就要想清楚什么时候步数才一定要加一呢?
贪心的思路,局部最优:当前可移动距离尽可能多走,如果还没到终点,步数再加一。整体最优:一步尽可能多走,从而达到最小步数。
思路虽然是这样,但在写代码的时候还不能真的能跳多远就跳多远,那样就不知道下一步最远能跳到哪里了。
所以真正解题的时候,要从覆盖范围出发,不管怎么跳,覆盖范围内一定是可以跳到的,以最小的步数增加覆盖范围,覆盖范围一旦覆盖了终点,得到的就是最小步数!
这里需要统计两个覆盖范围,当前这一步的最大覆盖和下一步最大覆盖。
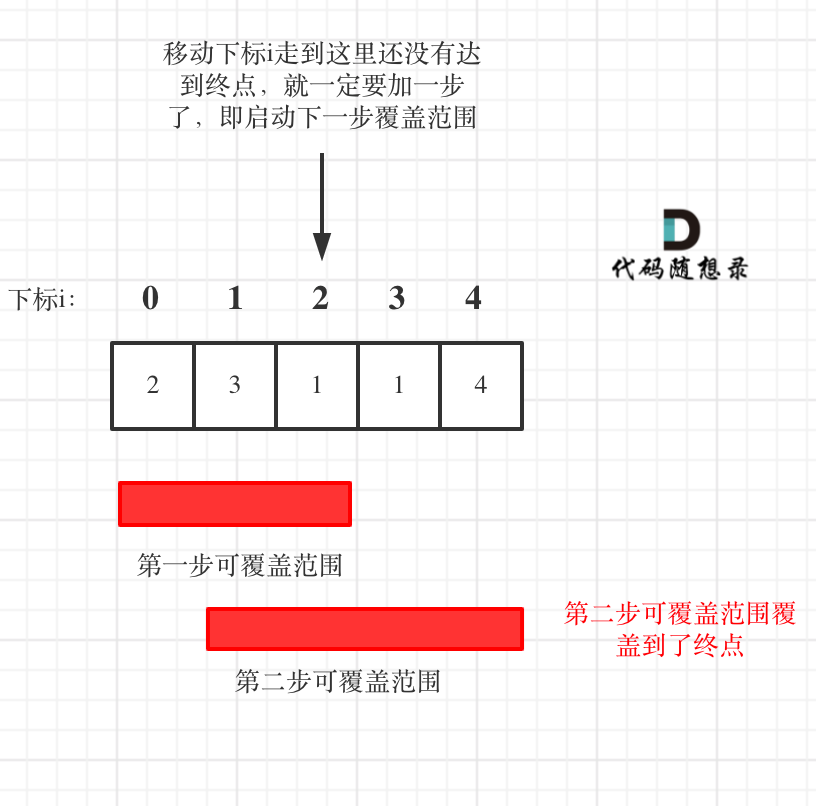
如果移动下标达到了当前这一步的最大覆盖最远距离了,还没有到终点的话,那么就必须再走一步来增加覆盖范围,直到覆盖范围覆盖了终点。
如图:
图中覆盖范围的意义在于,只要红色的区域,最多两步一定可以到!(不用管具体怎么跳,反正一定可以跳到)
从图中可以看出来,就是移动下标达到了当前覆盖的最远距离下标时,步数就要加一,来增加覆盖距离。最后的步数就是最少步数。
这里还是有个特殊情况需要考虑,当移动下标达到了当前覆盖的最远距离下标时
- 如果当前覆盖最远距离下标不是是集合终点,步数就加一,还需要继续走。
- 如果当前覆盖最远距离下标就是是集合终点,步数不用加一,因为不能再往后走了。
C++代码如下:(详细注释)
// 版本一
class Solution {
public:
int jump(vector<int>& nums) {
if (nums.size() == 1) return 0;
int curDistance = 0; // 当前覆盖最远距离下标
int ans = 0; // 记录走的最大步数
int nextDistance = 0; // 下一步覆盖最远距离下标
for (int i = 0; i < nums.size(); i++) {
nextDistance = max(nums[i] + i, nextDistance); // 更新下一步覆盖最远距离下标
if (i == curDistance) { // 遇到当前覆盖最远距离下标
if (curDistance != nums.size() - 1) { // 如果当前覆盖最远距离下标不是终点
ans++; // 需要走下一步
curDistance = nextDistance; // 更新当前覆盖最远距离下标(相当于加油了)
if (nextDistance >= nums.size() - 1) break; // 下一步的覆盖范围已经可以达到终点,结束循环
} else break; // 当前覆盖最远距离下标是集合终点,不用做ans++操作了,直接结束
}
}
return ans;
}
};依然是贪心,思路和方法一差不多,代码可以简洁一些。
针对于方法一的特殊情况,可以统一处理,即:移动下标只要遇到当前覆盖最远距离的下标,直接步数加一,不考虑是不是终点的情况。
想要达到这样的效果,只要让移动下标,最大只能移动到nums.size - 2的地方就可以了。
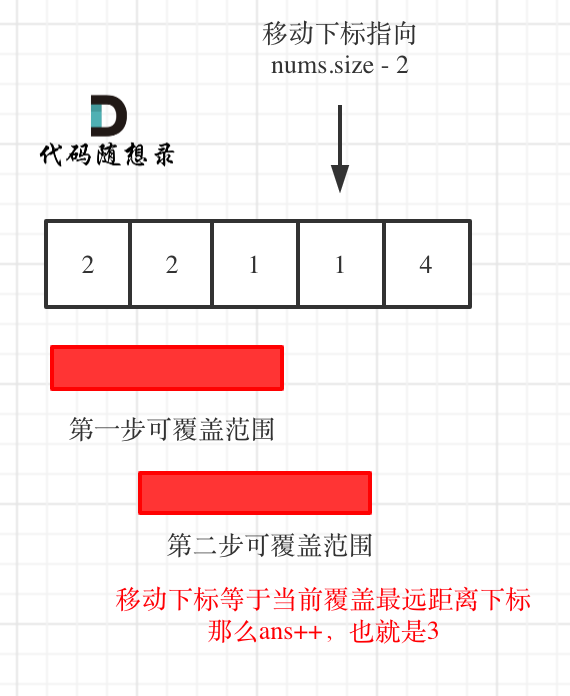
因为当移动下标指向nums.size - 2时:
-
如果移动下标等于当前覆盖最大距离下标, 需要再走一步(即ans++),因为最后一步一定是可以到的终点。(题目假设总是可以到达数组的最后一个位置),如图:
-
如果移动下标不等于当前覆盖最大距离下标,说明当前覆盖最远距离就可以直接达到终点了,不需要再走一步。如图:
代码如下:
// 版本二
class Solution {
public:
int jump(vector<int>& nums) {
int curDistance = 0; // 当前覆盖的最远距离下标
int ans = 0; // 记录走的最大步数
int nextDistance = 0; // 下一步覆盖的最远距离下标
for (int i = 0; i < nums.size() - 1; i++) { // 注意这里是小于nums.size() - 1,这是关键所在
nextDistance = max(nums[i] + i, nextDistance); // 更新下一步覆盖的最远距离下标
if (i == curDistance) { // 遇到当前覆盖的最远距离下标
curDistance = nextDistance; // 更新当前覆盖的最远距离下标
ans++;
}
}
return ans;
}
};可以看出版本二的代码相对于版本一简化了不少!
其精髓在于控制移动下标i只移动到nums.size() - 2的位置,所以移动下标只要遇到当前覆盖最远距离的下标,直接步数加一,不用考虑别的了。
相信大家可以发现,这道题目相当于55.跳跃游戏难了不止一点。
但代码又十分简单,贪心就是这么巧妙。
理解本题的关键在于:以最小的步数增加最大的覆盖范围,直到覆盖范围覆盖了终点,这个范围内最小步数一定可以跳到,不用管具体是怎么跳的,不纠结于一步究竟跳一个单位还是两个单位。
// 版本一
class Solution {
public int jump(int[] nums) {
if (nums == null || nums.length == 0 || nums.length == 1) {
return 0;
}
//记录跳跃的次数
int count=0;
//当前的覆盖最大区域
int curDistance = 0;
//最大的覆盖区域
int maxDistance = 0;
for (int i = 0; i < nums.length; i++) {
//在可覆盖区域内更新最大的覆盖区域
maxDistance = Math.max(maxDistance,i+nums[i]);
//说明当前一步,再跳一步就到达了末尾
if (maxDistance>=nums.length-1){
count++;
break;
}
//走到当前覆盖的最大区域时,更新下一步可达的最大区域
if (i==curDistance){
curDistance = maxDistance;
count++;
}
}
return count;
}
}// 版本二
class Solution {
public int jump(int[] nums) {
int result = 0;
// 当前覆盖的最远距离下标
int end = 0;
// 下一步覆盖的最远距离下标
int temp = 0;
for (int i = 0; i <= end && end < nums.length - 1; ++i) {
temp = Math.max(temp, i + nums[i]);
// 可达位置的改变次数就是跳跃次数
if (i == end) {
end = temp;
result++;
}
}
return result;
}
}class Solution:
def jump(self, nums: List[int]) -> int:
if len(nums) == 1: return 0
ans = 0
curDistance = 0
nextDistance = 0
for i in range(len(nums)):
nextDistance = max(i + nums[i], nextDistance)
if i == curDistance:
if curDistance != len(nums) - 1:
ans += 1
curDistance = nextDistance
if nextDistance >= len(nums) - 1: break
return ans# 贪心版本二
class Solution:
def jump(self, nums: List[int]) -> int:
if len(nums) == 1:
return 0
curDistance, nextDistance = 0, 0
step = 0
for i in range(len(nums)-1):
nextDistance = max(nextDistance, nums[i]+i)
if i == curDistance:
curDistance = nextDistance
step += 1
return step// 贪心版本一
func jump(nums []int) int {
n := len(nums)
if n == 1 {
return 0
}
cur, next := 0, 0
step := 0
for i := 0; i < n; i++ {
next = max(nums[i]+i, next)
if i == cur {
if cur != n-1 {
step++
cur = next
if cur >= n-1 {
return step
}
} else {
return step
}
}
}
return step
}
func max(a, b int) int {
if a > b {
return a
}
return b
}// 贪心版本二
func jump(nums []int) int {
n := len(nums)
if n == 1 {
return 0
}
cur, next := 0, 0
step := 0
for i := 0; i < n-1; i++ {
next = max(nums[i]+i, next)
if i == cur {
cur = next
step++
}
}
return step
}
func max(a, b int) int {
if a > b {
return a
}
return b
}var jump = function(nums) {
let curIndex = 0
let nextIndex = 0
let steps = 0
for(let i = 0; i < nums.length - 1; i++) {
nextIndex = Math.max(nums[i] + i, nextIndex)
if(i === curIndex) {
curIndex = nextIndex
steps++
}
}
return steps
};function jump(nums: number[]): number {
const length: number = nums.length;
let curFarthestIndex: number = 0,
nextFarthestIndex: number = 0;
let curIndex: number = 0;
let stepNum: number = 0;
while (curIndex < length - 1) {
nextFarthestIndex = Math.max(nextFarthestIndex, curIndex + nums[curIndex]);
if (curIndex === curFarthestIndex) {
curFarthestIndex = nextFarthestIndex;
stepNum++;
}
curIndex++;
}
return stepNum;
};object Solution {
def jump(nums: Array[Int]): Int = {
if (nums.length == 0) return 0
var result = 0 // 记录走的最大步数
var curDistance = 0 // 当前覆盖最远距离下标
var nextDistance = 0 // 下一步覆盖最远距离下标
for (i <- nums.indices) {
nextDistance = math.max(nums(i) + i, nextDistance) // 更新下一步覆盖最远距离下标
if (i == curDistance) {
if (curDistance != nums.length - 1) {
result += 1
curDistance = nextDistance
if (nextDistance >= nums.length - 1) return result
} else {
return result
}
}
}
result
}
}//版本一
impl Solution {
fn max(a: i32, b:i32) -> i32 {
if a > b { a } else { b }
}
pub fn jump(nums: Vec<i32>) -> i32 {
if nums.len() == 0 { return 0; }
let mut cur_distance: i32 = 0;
let mut ans: i32 = 0;
let mut next_distance: i32 = 0;
for i in 0..nums.len() {
next_distance = Self::max(nums[i] + i as i32, next_distance);
if i as i32 == cur_distance {
if cur_distance != (nums.len() - 1) as i32 {
ans += 1;
cur_distance = next_distance;
if next_distance == (nums.len() - 1) as i32 { break; }
}
else { break; }
}
}
ans
}
}//版本二
impl Solution {
fn max(a: i32, b:i32) -> i32 {
if a > b { a } else { b }
}
pub fn jump(nums: Vec<i32>) -> i32 {
let mut cur_distance: i32 = 0;
let mut ans: i32 = 0;
let mut next_distance: i32 = 0;
for i in 0..nums.len() - 1 {
next_distance = Self::max(nums[i] + i as i32, next_distance);
if i as i32 == cur_distance {
cur_distance = next_distance;
ans += 1;
}
}
ans
}
}