Inspired by Matt Parker's video on fair sharing, I decided to play with the concept some more and generate the sequence as well as to get familiar with matplotlib and numpy.
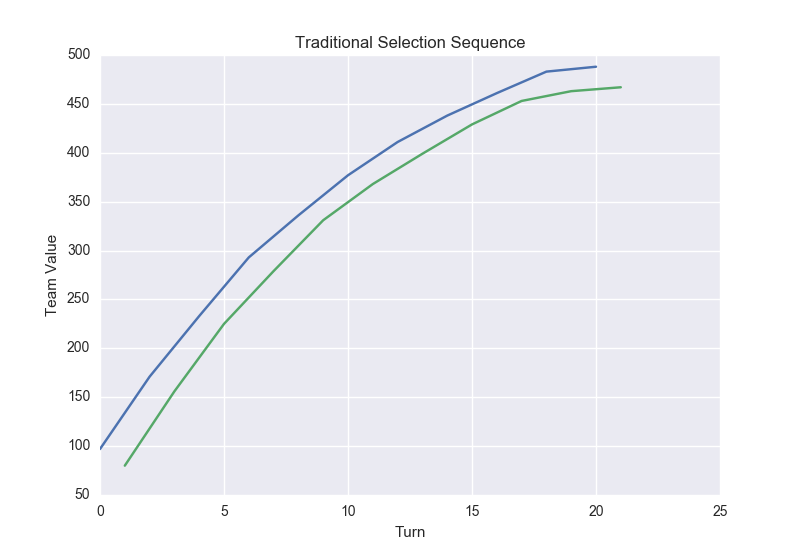
Flashback to recess time during elementary school. It's time for the two team captains to each select 9 other players from a pool of 18 to begin a friendly dodgeball game. Rock-paper-scissors for who picks first and you get seleted second. No biggie right? Your friend/captain got the second best person while the opponent is relegated to the third best. Come game time you can't help but feel a strange feeling in the back of your mind. Overall, the opponent team is better.
That's because if captain A gets to pick
[1, 3, 5, 7, 9, 11, 13, 15, 17, 19]
then captain B is forced to choose
[2, 4, 6, 8, 10, 12, 14, 16, 18, 20]
The average rank of team A is 100 while the average rank of team B is 110. Captain A consistently gets to pick the better of two players every round.
Using the Thue-Morse sequence, a 'fairer' outcome is possible by alternating captain A and B's turn order. This changes the skillgap of the teams from 1 to 0.2.